Using your Head is Permitted

Mesh animals are close relatives of their more famous cousins, the grid animals. If you ever played Tetris, you've had your share of trying to tile a grid using grid animals of size four. In the case of mesh animals, it isn't the grid that is being tiled, but rather the lattice that holds it.

To make the whole thing more concrete, consider the following mesh animal:

It can be used, for example, to tile 2-by-2 and 3-by-3 squares as shown:

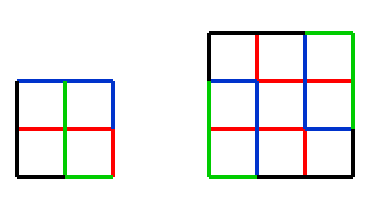
As can be seen in the example, we are interested in tiling an entire square mesh by duplicates of the same mesh animal, where this animal can appear in any position, any orientation, and possibly also mirror-reversed.

The riddle this month will be composed of two parts. Answer either or both parts for your name to be mentioned as a solver. A separate list of solvers will be kept for each of the two parts.

Part 1:

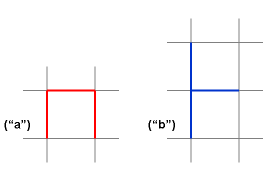
The riddle should be solved separately for each animal, of course.

Prove your answer.

(Readers are welcome to try and solve for non-square rectangles, as well. The solution to the extended riddle will appear with the rest of this riddle's solution. Nevertheless, only squares are required to solve Part 1.)

Part 2:

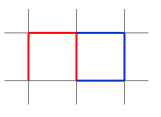
A tiling is called connected if there is a path of connected mesh animal pairs that links any two mesh animals in the tiling. (This can be thought of as a form of graph connectivity.)

The question is: what is the largest square that can be tiled by this mesh animal, by use of a connected tiling?

Prove your answer.

Readers wishing for a greater challenge are welcome to try and solve the question of which squares and rectangles can be tiled by the mesh animal given in the first example (for which 2x2 and 3x3 square-tilings were demonstrated). The solution for squares will appear with the answer to this month's riddle. However, you are not requested to send answers for this.

List of solvers:

Part 1:

Part 2: